Undergraduate Students Research Projects

UNDERGRADUATE STUDENTS RESEARCH PROJECTS
Analysis of coating and rimming thin-film flows

The time evolution of the height of a liquid film which spreads over a solid surface under the action of the surface tension and viscosity can be described by lubrication models. These models have been shown to be extremely useful approximations to the full Navier-Stokes equations that appear in the study of motion and instabilities of contact lines in liquid films dynamics. Thin fluid films can generate fascinating behaviors: “fingering” phenomena, periodic waves, shock and fronts, regular and chaotic structure formations.

If one rotates a horizontal cylinder about its axis, then depending on the volume of the fluid,the fluid and surface properties, the speed of rotation and the strength of gravity, a fluid on the outside of the cylinder may coat the cylinder surface (coating flow). Similarly, a fluid on the inside of the cylinder may coat the inner surface (rimming flow). In both situations, interesting structures and dynamics can be observed experimentally and computationally.Suppose a small volume of liquid is placed inside a horizontally positioned cylinder and it is not rotating, the puddle would sit symmetrically in the bottom of the cylinder due to the gravity field (potential energy is minimized). Slow rotation of the cylinder in an anticlockwise direction would cause some liquid to be entrained. The symmetric puddle would transform into a shockwave-like structure near the rising side of the cylinder. For slow rotation rates these shock waves are experimentally observable and stable with respect to a small tilt of the cylinder.

2012-2013, (co-supervision with Chiu-Yen Kao) ) Sarun Seepun ((Pitzer College, 3rd year undergraduate student)

The individual study project “Mathematics of thin-film flows”.

We study an initial-boundary value problem for a fourth-order parabolic partial differential equation with an unknown potential. The equation originated from the linearization of a two-dimensional Couette flow model, that was recently proposed by Benilov and Vynnycky. In the case of a 180 degree contact angle between liquid and a moving plate Benilov and Vynnycky conjectured that the speed of the contact line blows up to infinity in finite time. We show that depending on the initial data and parameter values two different regimes can be observed. The speed of the contact line may blow up to infinity or converge to a constant.

2010-2011, (co-supervision with Stephen Morris, Mary Pugh) Gary Yan (UofT, 3rd year undergraduate student, Engineering Science Department)

The laboratory project “Experimental study of rimming flow steady states for low rotation speed regime”.

A number of different phenomena seen in the free-surface flow inside a partially filled circular cylinder which is rotated about its horizontal axis of symmetry. At low angular velocities the flow settles into a steady two-dimensional flow with a front where the coating film coalesces with the pool at the bottom of the cylinder. This mode becomes unstable at higher angular velocities, initially to a sloshing mode on the rising side of the coating film and then to an axial instability on the front. At still higher angular velocities and volume fractions, a number of different inertial instabilities and patterns appear. Gary will study experimentally rimming flows in rotating cylinders of different diameters.

2010(Summer), MITACS-Fields Undergraduate Summer Research Program, (co-supervision with Dmitry Pelinovsky ) Alex Kulyk (Kharkiv Polytechnic Institute, Ukraine, 3rd year undergraduate student, Department of Applied Mathematics), Daniel Badali (UofT, 4th year undergraduate student, Department of Physics), Steven Pollack (McGill University, 3rd year undergraduate student, Department of Mathematics).

The research project: “Bifurcation analysis of coating flow steady states for low surface tension regime”.

With students we analyzed numerically existence and stability of smoothed-shock steady states ( discontinuous steady state solutions of Moffatt equations exist for the critical flux value 2/3) of rimming flows for small surface tension regime. Steven coded the “turning point algorithm” to overcome sharp turning points on the bifurcation diagram (Matlab). We confirmed numerically saddle-node bifurcations. Got beyond Benilov’s result (he found 3 steady states with the same mass), Steven’s code found 5 steady states with the same mass.

2010 (Summer), NSERC Undergraduate Summer Research, (co-supervision with Almut Burchard, Gideon Simpson) Dan Ginsberg (UofT, 3rd year undergraduate student, Department of Mathematics).

The research project: “Strong positivity property of weak steady states in coating flows”.

Dan proved strong positivity of generalized strong steady state solutions for some fourth order degenerate parabolic equation. His proof is based on a construction of test functions and on applications of local entropy inequalities. He also helped develop and implement a robust algorithm for computing these steady state solutions using Newton’s method and two parameter continuation algorithm.

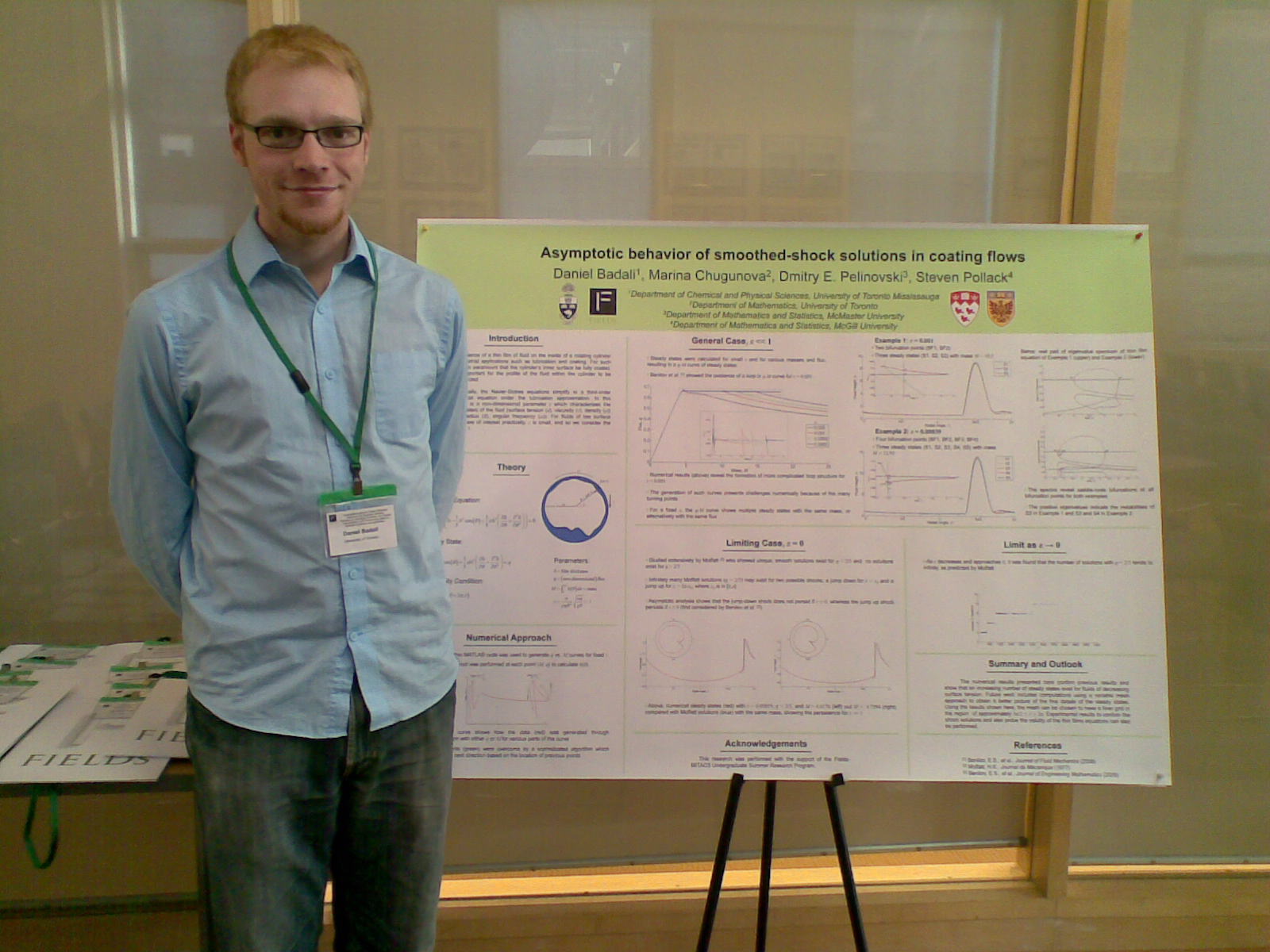
(November 1-5, 2010) Workshop on Geometric Probability and Optimal Transportation ( Organizers B. Klartag and R. McCann ), Fields Institute.

Poster presentation: ” Asymptotic behavior of smoothed-shock solutions in coating flows” by Daniel Badali (UofT, 4th year undergraduate student, Department of Physics),

The behavior of thin film of fluid on the inside of a rotating cylinder is examined. The physical parameters of the fluid (surface tension, viscosity, etc.) as well as the radius and angular frequency of the cylinder can be represented by the non-dimensional parameter e. For fluids of low surface tension, which are of interest practically, e is small, and so we consider the case when e << 1. Numerical results reveal the existence of an increasing number of steady states for e values down to 0.001, which is an order of magnitude smaller than previously published results.

2009 (Summer), NSERC Undergraduate Summer Research, (co-supervision with Almut Burchard) Guo Weiwei (UofT, 4th year undergraduate student, Department of Mathematics).

The research project: ” Numerical analysis of spectral stability of steady states in rimming flows “.

Guo developed a robust algorithm for computing eigenvalues and eigenfunctions of a non-self-adjoint operator using shooting method and implemented it in Matlab code.

He currently works in the Model Vetting group within the Market and Model Risk Management division at Manulife Financial. His work involves testing and validation of the financial pricing and risk models.